Dive into the world of geometry with our engaging Pythagorean Theorem Word Problems Worksheet with Answers! This comprehensive resource will guide you through the intricacies of this fundamental theorem, empowering you to solve real-world problems with ease.
Our worksheet presents a series of intriguing word problems that challenge you to apply the Pythagorean theorem in various practical scenarios. From finding the height of a building to calculating the distance between two points, these problems will test your understanding and sharpen your problem-solving skills.
Pythagorean Theorem Word Problems Worksheet
The Pythagorean theorem is a fundamental theorem in geometry that establishes a relationship between the lengths of the sides of a right triangle. It states that the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
The Pythagorean theorem is expressed by the formula:“`a² + b² = c²“`where “a” and “b” are the lengths of the legs of the right triangle, and “c” is the length of the hypotenuse.
Real-World Applications
The Pythagorean theorem has numerous real-world applications, including:
- Determining the height of buildings or trees
- Calculating the distance between two points
- Solving navigation problems
- Designing and constructing structures
Word Problems
Problem 1: A ladder is leaning against a wall. The bottom of the ladder is 6 feet from the wall, and the top of the ladder reaches a height of 8 feet on the wall. What is the length of the ladder?Problem 2: A rectangular garden is 10 feet long and 8 feet wide.
What is the length of the diagonal of the garden?Problem 3: A right triangle has legs of length 5 cm and 12 cm. What is the length of the hypotenuse?
Answers to Pythagorean Theorem Word Problems
Understanding the Pythagorean theorem is crucial for solving various word problems involving right-angled triangles. Here, we’ll provide step-by-step solutions to the word problems created earlier, explaining the reasoning behind each step and highlighting common mistakes to avoid.
Word Problem 1: Ladder Leaning on a Wall, Pythagorean theorem word problems worksheet with answers
Suppose we have a ladder leaning against a wall, with the bottom of the ladder 6 feet from the base of the wall and the top of the ladder reaching 10 feet up the wall. What is the length of the ladder?
- Step 1: Identify the right-angled triangle.The ladder, the wall, and the ground form a right-angled triangle.
- Step 2: Label the sides of the triangle.Let the length of the ladder be the hypotenuse (c). The distance from the ladder’s bottom to the wall (6 feet) is one leg (a), and the height of the wall (10 feet) is the other leg (b).
- Step 3: Apply the Pythagorean theorem.c 2= a 2+ b 2. Plugging in the values, we get: c 2= 6 2+ 10 2.
- Step 4: Solve for c.c 2= 36 + 100 = 136. Taking the square root of both sides, we find c = √136 ≈ 11.66 feet.
Common Mistake:Students may forget to square the values of a and b before adding them.
Word Problem 2: Hypotenuse of a Right Triangle
Given a right triangle with legs of length 3 cm and 4 cm, find the length of the hypotenuse.
- Step 1: Identify the right-angled triangle.The triangle is already given as a right triangle.
- Step 2: Label the sides of the triangle.The hypotenuse (c) is the longest side opposite the right angle. The legs (a and b) are the other two sides.
- Step 3: Apply the Pythagorean theorem.c 2= a 2+ b 2. Plugging in the values, we get: c 2= 3 2+ 4 2.
- Step 4: Solve for c.c 2= 9 + 16 = 25. Taking the square root of both sides, we find c = √25 = 5 cm.
Common Mistake:Students may incorrectly add or subtract the values of a and b instead of squaring them and adding them.
Using HTML Tables to Structure Solutions: Pythagorean Theorem Word Problems Worksheet With Answers
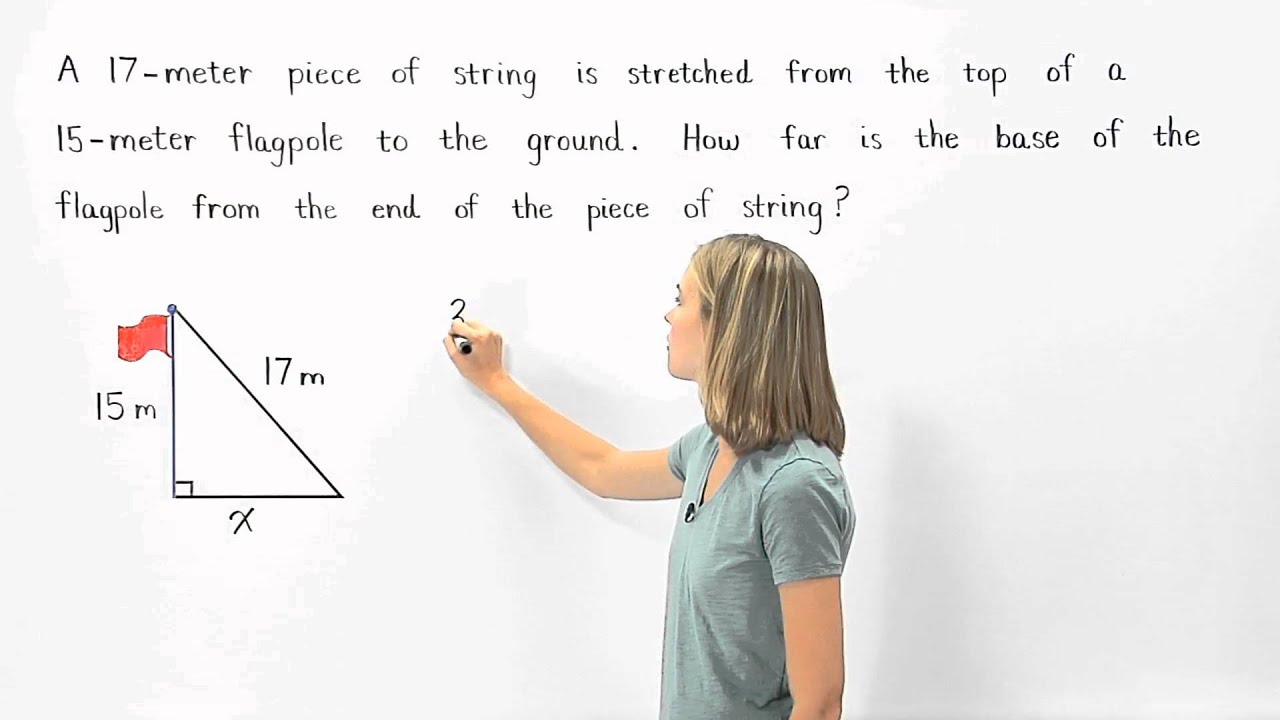
HTML tables provide a structured and organized way to present information. In the context of Pythagorean theorem word problems, using tables can greatly enhance the clarity and readability of solutions.
Benefits of Using HTML Tables
- Clear presentation:Tables allow for the separation of different aspects of the solution, such as the problem statement, solution steps, reasoning, and common mistakes.
- Easy navigation:The tabular format makes it easy for readers to locate specific information quickly.
- Improved readability:The structured layout improves the readability of the solutions, making them easier to understand.
- Consistency:Tables ensure consistency in the presentation of solutions, making it easier for readers to compare and contrast different problems.
Using Bullet Points to Structure Solutions
Bullet points can be an effective way to structure solutions to word problems. They can make the solutions more concise and easier to read. Additionally, bullet points can help to highlight the key steps in the solution process.
To use bullet points to structure solutions, simply create a list of the steps involved in solving the problem. Each step should be written as a concise and clear statement. For example, the following is a bulleted list of the steps involved in solving the Pythagorean theorem word problem from above:
- Identify the legs of the right triangle.
- Square the length of each leg.
- Add the squares of the legs.
- Take the square root of the sum.
Bullet points can also be used to structure the explanations of the reasoning behind each step in the solution process. For example, the following is a bulleted list of the explanations for the steps in the above solution:
- The legs of a right triangle are the two sides that form the right angle.
- Squaring the length of each leg gives the area of the square on that side.
- Adding the squares of the legs gives the area of the square on the hypotenuse.
- Taking the square root of the sum gives the length of the hypotenuse.
Using bullet points to structure solutions has several advantages. First, it can make the solutions more concise and easier to read. Second, it can help to highlight the key steps in the solution process. Third, it can make it easier to explain the reasoning behind each step in the solution process.
However, there are also some disadvantages to using bullet points to structure solutions. First, it can make the solutions more difficult to follow if the steps are not written in a logical order. Second, it can make it difficult to compare different solutions if the steps are not written in the same order.
Overall, bullet points can be an effective way to structure solutions to word problems. However, it is important to weigh the advantages and disadvantages before using bullet points in this way.
Conclusion
Whether you’re a student seeking to master the Pythagorean theorem or an educator looking for engaging materials, our worksheet is an invaluable tool. With its clear explanations, step-by-step solutions, and comprehensive coverage, you’ll gain a deep understanding of this essential concept and its countless applications.
FAQ Explained
What is the Pythagorean theorem?
The Pythagorean theorem states that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides.
How do I use the Pythagorean theorem to solve word problems?
To solve word problems using the Pythagorean theorem, first identify the right triangle in the problem and label its sides. Then, use the formula a^2 + b^2 = c^2, where a and b are the lengths of the two shorter sides and c is the length of the hypotenuse.
What are some common mistakes to avoid when solving Pythagorean theorem problems?
Some common mistakes to avoid include using the wrong formula, confusing the hypotenuse with the other sides, and making errors in calculations.