With “A Large One Might Have More Sides” as the captivating title, embark on a fascinating journey into the world of geometric shapes and polygons. This engaging intro will pique your curiosity, leading you through a delightful exploration of their types, properties, and examples.

Let’s dive into the intricate world of regular and irregular polygons, discovering their unique characteristics and how they differ from one another. We’ll also delve into the fascinating realm of polyhedra, examining their defining features and classification based on the number of faces.

Prepare to be amazed by the wonders of geometry!

Geometric Shapes

Geometric shapes are two-dimensional figures that are defined by their specific properties and characteristics. These shapes have distinct attributes, such as number of sides, angles, and lengths, that differentiate them from other shapes. Understanding geometric shapes is fundamental in various fields, including mathematics, architecture, design, and engineering.

Types of Geometric Shapes

There are several types of geometric shapes, each with its own unique characteristics. Some of the most common types include:

• Triangles:Triangles are polygons with three sides. They can be classified based on the length of their sides (equilateral, isosceles, or scalene) or the measurement of their angles (acute, right, obtuse).
• Quadrilaterals:Quadrilaterals are polygons with four sides. Examples of quadrilaterals include squares, rectangles, parallelograms, and trapezoids.
• Circles:Circles are perfectly round shapes with no straight sides. They are defined by a center point and a radius, which is the distance from the center to any point on the circumference.
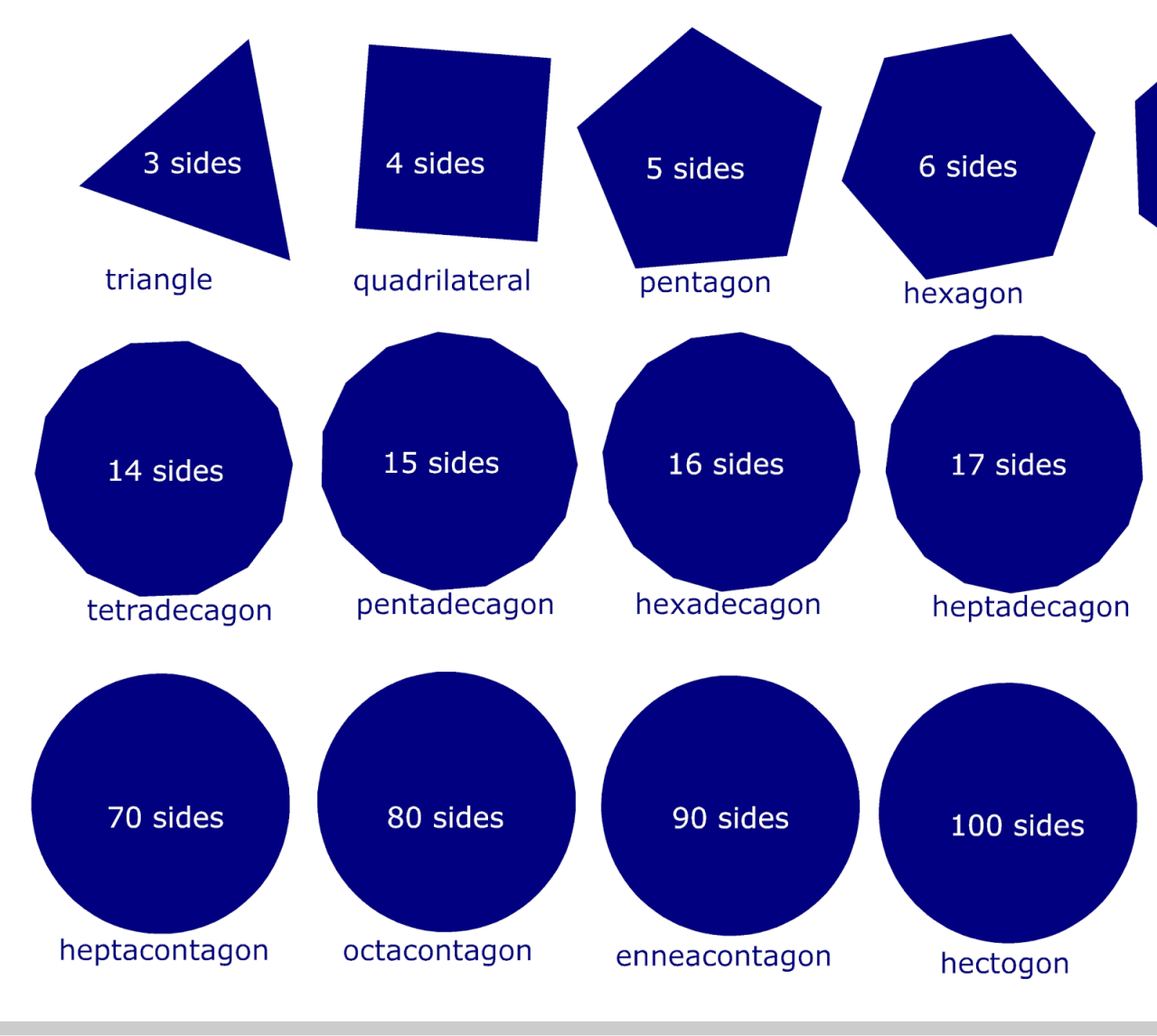
• Polygons:Polygons are closed shapes with three or more straight sides. Examples of polygons include pentagons, hexagons, and octagons.

Characteristics of Geometric Shapes

Geometric shapes possess specific characteristics that distinguish them from one another. These characteristics include:

• Number of Sides:Each shape has a specific number of sides, which can range from three (in triangles) to an infinite number of sides (in circles).
• Angles:Geometric shapes have different angles between their sides. For example, triangles have three angles, while quadrilaterals have four.
• Lengths:The lengths of the sides of a geometric shape can be equal or different, depending on the type of shape. For instance, squares have equal side lengths, while rectangles have opposite sides of equal length.
• Symmetry:Some geometric shapes exhibit symmetry, which means that they can be divided into two equal halves that mirror each other. Examples of symmetric shapes include circles and regular polygons.

Polygons

Polygons are two-dimensional shapes that are formed by connecting straight line segments. These line segments are called sides, and the points where the sides intersect are called vertices. Polygons can have different numbers of sides, ranging from three to infinity.Polygons

have several properties that distinguish them from other shapes. Firstly, the sum of the interior angles of a polygon always adds up to a specific value. The formula to calculate the sum of the interior angles of a polygon is (n-2)

180 degrees, where n represents the number of sides of the polygon.

Another property of polygons is that all of their sides are straight lines. This means that the sides do not curve or bend. Additionally, all the sides of a polygon have the same length, except in irregular polygons where the sides can have different lengths.Polygons

can be classified based on the number of sides they have. Here are some examples of polygons with different numbers of sides:

Triangle

A triangle is a polygon with three sides. It is the simplest polygon and is formed by connecting three line segments. Triangles can be classified further based on the lengths of their sides and the measures of their angles.

Quadrilateral

A quadrilateral is a polygon with four sides. It is formed by connecting four line segments. Examples of quadrilaterals include squares, rectangles, parallelograms, and trapezoids.

Pentagon

A pentagon is a polygon with five sides. It is formed by connecting five line segments. Regular pentagons have all sides and angles equal, while irregular pentagons have sides and angles of different lengths and measures.

Hexagon

A hexagon is a polygon with six sides. It is formed by connecting six line segments. Hexagons can be regular or irregular, depending on the equality of their sides and angles.

Heptagon

A heptagon is a polygon with seven sides. It is formed by connecting seven line segments. Regular heptagons have all sides and angles equal, while irregular heptagons have sides and angles of different lengths and measures.

Octagon

An octagon is a polygon with eight sides. It is formed by connecting eight line segments. Octagons can be regular or irregular, depending on the equality of their sides and angles.

Nonagon, A large one might have more sides

A nonagon is a polygon with nine sides. It is formed by connecting nine line segments. Regular nonagons have all sides and angles equal, while irregular nonagons have sides and angles of different lengths and measures.

Decagon

A decagon is a polygon with ten sides. It is formed by connecting ten line segments. Decagons can be regular or irregular, depending on the equality of their sides and angles.These are just a few examples of polygons with different numbers of sides.

Polygons play an important role in various fields, including geometry, architecture, and computer graphics.

Regular Polygons

Regular polygons are polygons that have equal side lengths and equal interior angles. In other words, all sides and angles of a regular polygon are congruent.Regular polygons have several properties that distinguish them from other polygons. Firstly, all sides of a regular polygon are of equal length.

This means that if we measure any two sides of a regular polygon, they will be the same length. Secondly, all interior angles of a regular polygon are also equal. This means that if we measure any two interior angles of a regular polygon, they will be the same measure.The

relationship between the number of sides and the angles in regular polygons is determined by a simple formula. To find the measure of each interior angle in a regular polygon, we use the formula: Interior angle = (n

• 2)
• 180 / n

where n represents the number of sides of the regular polygon. This formula allows us to find the measure of each interior angle in any regular polygon, regardless of the number of sides.Now, let’s take a look at some examples of regular polygons with different numbers of sides:

1. Triangle (3 sides)

A triangle is the simplest regular polygon, with three equal sides and three equal interior angles measuring 60 degrees each.

2. Square (4 sides)

A square is a regular polygon with four equal sides and four equal interior angles measuring 90 degrees each.

3. Pentagon (5 sides)

A pentagon is a regular polygon with five equal sides and five equal interior angles measuring 108 degrees each.

4. Hexagon (6 sides)

A hexagon is a regular polygon with six equal sides and six equal interior angles measuring 120 degrees each.

5. Heptagon (7 sides)

A heptagon is a regular polygon with seven equal sides and seven equal interior angles measuring approximately 128.57 degrees each.These examples demonstrate how the number of sides in a regular polygon affects the measure of its interior angles. As the number of sides increases, the measure of each interior angle also increases.

Irregular Polygons

Irregular polygons, as the name suggests, are polygons that do not have equal side lengths or equal angle measures. Unlike regular polygons, irregular polygons have sides and angles that vary throughout their shape.

Here are some properties of irregular polygons:

• Irregular polygons have sides of different lengths.
• The interior angles of irregular polygons are not congruent.
• Irregular polygons do not have any lines of symmetry.
• The exterior angles of irregular polygons are also not congruent.
• The sum of the interior angles of an irregular polygon is always equal to (n-2) – 180 degrees, where n is the number of sides.

Irregular polygons differ from regular polygons in that regular polygons have equal side lengths and equal angle measures throughout their shape. Regular polygons are symmetrical and have multiple lines of symmetry. On the other hand, irregular polygons lack symmetry and have varying side lengths and angle measures.

Examples of Irregular Polygons

Irregular polygons can have different numbers of sides. Here are some examples:

• Triangle: A triangle is an irregular polygon with three sides and three angles.
• Pentagon: A pentagon is an irregular polygon with five sides and five angles.
• Hexagon: A hexagon is an irregular polygon with six sides and six angles.
• Heptagon: A heptagon is an irregular polygon with seven sides and seven angles.
• Octagon: An octagon is an irregular polygon with eight sides and eight angles.

These examples demonstrate how irregular polygons can have different numbers of sides, with each side and angle varying in length and measure.

Polyhedra

Polyhedra are three-dimensional geometric figures that are made up of flat faces, straight edges, and sharp vertices. The term “polyhedra” is derived from the Greek words “poly,” meaning “many,” and “hedra,” meaning “faces.” Therefore, polyhedra are objects with many faces.Polyhedra

have several characteristics that distinguish them from other geometric figures. Firstly, all the faces of a polyhedron are polygons. These polygons can be of any shape, including triangles, rectangles, pentagons, or hexagons. Secondly, the edges of a polyhedron are straight line segments where two faces intersect.

Finally, the vertices of a polyhedron are the points where three or more edges meet.Polyhedra can be classified based on the number of faces they have. There are three main categories: convex polyhedra, concave polyhedra, and star polyhedra. Convex polyhedra have all their faces as convex polygons, meaning that all their internal angles are less than 180 degrees.

Concave polyhedra have at least one concave face, where at least one internal angle is greater than 180 degrees. Star polyhedra have at least one face that is not a polygon, but rather a star-shaped figure.Here are some examples of polyhedra with different numbers of faces:

• A tetrahedron is a polyhedron with four triangular faces.
• A cube is a polyhedron with six square faces.
• An octahedron is a polyhedron with eight triangular faces.
• A dodecahedron is a polyhedron with twelve pentagonal faces.
• An icosahedron is a polyhedron with twenty triangular faces.

Classification of Polyhedra based on the Number of Faces

Polyhedra can be further classified based on the number of faces they have. The classification is as follows:

1. Triangular polyhedra

These are polyhedra with only triangular faces. Examples include tetrahedrons and octahedrons.

2. Quadrilateral polyhedra

These are polyhedra with only quadrilateral faces. An example is a cube.

3. Pentagonal polyhedra

These are polyhedra with only pentagonal faces. An example is a dodecahedron.

4. Hexagonal polyhedra

These are polyhedra with only hexagonal faces. An example is an icosahedron.

5. Mixed polyhedra

These are polyhedra with a combination of different types of faces. Examples include prisms, pyramids, and antiprisms.It is important to note that there are many more polyhedra with different numbers of faces, but the above examples provide a good overview of the different classifications.

Regular Polyhedra: A Large One Might Have More Sides

Regular polyhedra are three-dimensional geometric shapes that have congruent regular polygons as their faces. Each face of a regular polyhedron is identical in shape and size, and the angles between the faces are also the same. The edges of a regular polyhedron are equal in length, and all vertices have the same number of edges meeting at them.Regular

polyhedra have several important properties. Firstly, they are convex, meaning that they have no indentations or holes. Secondly, their faces are congruent regular polygons, which means that all sides and angles of each face are equal. Thirdly, the angles between the faces of a regular polyhedron are also congruent.The

relationship between the number of sides, vertices, and faces in a regular polyhedron is given by Euler’s formula: F + V = E + 2, where F represents the number of faces, V represents the number of vertices, and E represents the number of edges.

For regular polyhedra, the number of faces (F), vertices (V), and edges (E) are related by the formula: F + V

E = 2.

There are five regular polyhedra, known as the Platonic solids. These include:

Tetrahedron

A regular polyhedron with 4 triangular faces, 4 vertices, and 6 edges.

Cube

A regular polyhedron with 6 square faces, 8 vertices, and 12 edges.

Octahedron

A regular polyhedron with 8 triangular faces, 6 vertices, and 12 edges.

Dodecahedron

A regular polyhedron with 12 pentagonal faces, 20 vertices, and 30 edges.

Icosahedron

A regular polyhedron with 20 triangular faces, 12 vertices, and 30 edges.These regular polyhedra have symmetric and balanced structures, making them fascinating objects of study in geometry.

Examples of Regular Polyhedra with Different Numbers of Sides

• Tetrahedron: 4 triangular faces
• Hexahedron (Cube): 6 square faces
• Octahedron: 8 triangular faces
• Dodecahedron: 12 pentagonal faces
• Icosahedron: 20 triangular faces

Irregular Polyhedra

An irregular polyhedron is a three-dimensional geometric shape that has irregular polygons as its faces. Unlike regular polyhedra, which have congruent regular polygons as faces, irregular polyhedra have faces that do not meet the criteria of regularity.

Irregular polyhedra possess several properties that distinguish them from regular polyhedra:

• Irregular polyhedra have faces that are not congruent or regular polygons.
• The angles between the faces of irregular polyhedra can vary.
• The edges of irregular polyhedra are not necessarily congruent.
• Irregular polyhedra do not have a fixed set of edge lengths or angles.

Examples of Irregular Polyhedra with Different Numbers of Sides

Irregular polyhedra can have varying numbers of sides. Here are some examples:

Tetrahedron

A tetrahedron is a four-sided irregular polyhedron. It has four triangular faces, and each vertex is connected to three edges.

Hexahedron (Cube)

A hexahedron, commonly known as a cube, is a six-sided irregular polyhedron. It has six square faces, and each vertex is connected to three edges.

Octahedron

An octahedron is an eight-sided irregular polyhedron. It has eight triangular faces, and each vertex is connected to four edges.

Dodecahedron

A dodecahedron is a twelve-sided irregular polyhedron. It has twelve pentagonal faces, and each vertex is connected to three edges.

Icosahedron

An icosahedron is a twenty-sided irregular polyhedron. It has twenty triangular faces, and each vertex is connected to five edges.

Epilogue

In conclusion, “A Large One Might Have More Sides” has taken us on an enthralling adventure through geometric shapes, polygons, and polyhedra. We’ve explored their diverse properties, classifications, and examples, unveiling the beauty and complexity of these mathematical wonders. Whether regular or irregular, these shapes and forms continue to inspire and fascinate us.

Keep exploring and discovering the intriguing world of geometry!

Top FAQs

What are some examples of irregular polygons?

Irregular polygons include shapes like a trapezoid, pentagon, and hexagon, where their sides and angles are not equal or congruent.

How do regular polygons differ from irregular polygons?

Regular polygons have congruent sides and angles, while irregular polygons have sides and angles that are not equal or congruent. Regular polygons also have a symmetrical appearance, unlike irregular polygons.

What are polyhedra and how are they classified?

Polyhedra are three-dimensional geometric figures with flat faces and straight edges. They are classified based on the number of faces they have. Examples include tetrahedrons, cubes, and dodecahedrons.